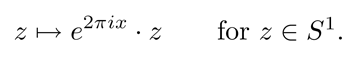
My research interests are in the theory of dynamical systems and and its connections with other branches of mathematics such as number theory, geometry and representation theory. By a dynamical system I mean a space equipped with a group of transformations. The orbit structure of these transformations is typically very complicated: there are orbits that fill the space densely or accumulate on an exotic fractal set. Nonetheless, one can develop techniques to analyse statistical properties of orbits over long time periods. This problem is not only of fundamental importance in the theory of dynamical systems, but also has striking applications to number theory. For instance, in the theory of Diophantine approximation one is interested in the best possible approximation for a given real number x by rational numbers. It turns out that this problem is intimately related to dynamical properties of the rotation of the circle S1:
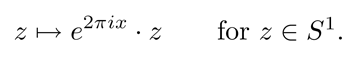
Another way to appoach this problem is to consider the action of the group SL(2,Z) on the real line by fractional linear transformations:
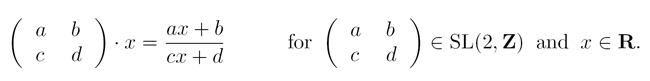
In both cases information about structure of orbits provides deep insights into Diophantine properties of real numbers.
Distribution of Orbits and Ergodic Theorems
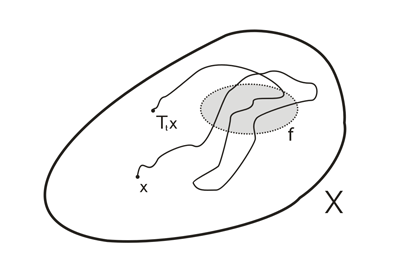
One of the fundamental problems in ergodic theory is to describe the asymptotic distribution of orbits. Let us consider a one-parameter family of transformations Tt of a space X. Given a point x in X one is interested in measuring the position of Ttx for large t. Usually it is impossible to evaluate the limit of Ttx preceisely, but one can make a statistical prediction as follows: take a test function f on X and consider the average
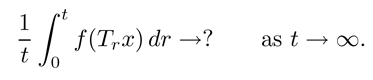
Roughly speaking, this average represents the "probability" that Ttx is in the "region" of space determined by f, i.e., its limit gives the asymptotic distribution of the orbit Ttx. For a one-parameter family of transformations and generic points x this distribution is determined by the the Birkhoff-von Neumann ergodic theorem.
Proving analogues of ergodic theorems for more general dynamical systems is an active area of research. See:
Ergodic theorems describe behaviour of generic orbits, but for many applications it is crucial to establish asymptotic distribution of a given orbit. Remarkable results in this direction has been established by Dani, Margulis, and Ratner, see:
These results lead to many far-reaching applications to number theory.
More generally, one is interested to understand finer statistical properties of the sequence of samples f(Ttx ) computed along orbits. For chaotic dynamical systems, one might expect that these samples are "quasi-independent" in a suitable sense. This lead to analysis of correlations and higher-order correlations:
For instance, one can show that the samples f(Ttx ) satisfy the Central Limit Theorem and other probabilistic limit laws, as sequences of independent random variables.
Dynamical Systems and Diophantine Geometry

Quite a few problems in number theory can be modelled using methods for the theory of dynamical systems. The picture on the left represents the set of rational points on the projective plane. What is the distribution of these points? More generally, given a system of polynomial equations, what can one say about the set of rational solutions? Although this is one of the oldest problems in mathematics, it is still out of reach in its full generality, but when the system equations has a large group of symmetries, one may be able to analyse the set of solutions using dynamical techniques.
One of the most remarkable applications of dynamical systems to number theory was discovered by G. Margulis. It was conjectured by Oppenheim in late 20's that in particular the following set of real numbers is dense:
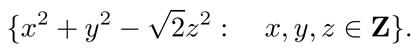
This is indeed the case, but it turns out to be surprisingly difficult to prove. In fact the Oppenheim conjecture was proved by G. Margulis only in 1987, and his proof uses dynamics on the space SL(3,R)/SL(3,Z).
There are many more surprising applications of dynamical
systems to number theory. If you would like to know more, you may consult
lecture notes from my courses:
or the following references:
Rigidity Phenomena in Dynamical Systems
Another direction of my research is various rigidity properties of groups and group actions. Consider, for instance, the problem of classification of isomorphisms of the group SL(n,Z). While the group SL(2,Z) has a large number of distinct isomorphisms, the situation is very different for n>2. It turns out that when n>2 every isomorphism of SL(n,Z) is the restriction of a algebraic isomorphism of the ambient group SL(n,R). This remarkable rigidity phenomenon was proved by G. Prasad, following previous work of G. Mostow (see Mostow rigidity).
An ambitious programme, proposed by Zimmer in 80's, is aimed at classification of actions of groups like SL(n,Z), n>2, on compact manifolds. It is believed that every such action is "built" from well-known algebraic actions of the group. More generally given a dynamical system one may ask, for instance, to describe
This programme has been actively pursued for several classes
of actions. For further readings, see my courses
and the following references: